
If you have ever stared at a snowflake, a tree’s branches or even the bronchi in a human lung – and wondered where the beautiful complexity of the pattern comes from, then this article is for you. If you have not, this is also for you: in this article, we will learn about their properties and explore some real-world appearances of these thought-provoking shapes.
Maths in fractals
What are fractals? In simple words, fractals are geometric shapes that exhibit self-similarity at different scales. Self-similarity, in colloquial terms, means that you could find similar patterns at different scales as you zoom in and out of the diagram. Here is an example: the “Koch curve”, also known as the “Koch snowflake”.

You could start creating this curve by dividing a straight line into three equal segments. Replace the middle segment by the two sides of an equilateral triangle of the same length as the segment removed. Now repeat, taking each of the four resulting segments, dividing them into three equal parts and replacing each of the middle segments by two sides of an equilateral triangle. If you repeat this operation enough times, you will obtain this shape:

The pattern at the edges, each of which resembles a half-opened hexagram, is very similar to the centre shape, which is also a large hexagram (imagine squinting – the sides would blur out).
Some interesting properties of the curve are its perimeter and its area. To find its perimeter, we first need to know the number of sides after n iterations. This is given by:
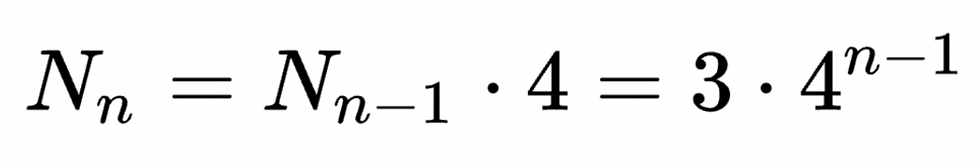
Therefore, if the original equilateral triangle has sides of length S, the length of each side of the snowflake after n iterations is:

As for how long a Koch curve could be, we could consider how each iteration creates four times as many segments as the previous shape, with each segment having one third of the length of each segment previously. Hence, the length of the curve after n iterations will be (4/3)^n times the original perimeter. As n tends to infinity, this number also tends to infinity.
Next, we could consider the area of the Koch curve. In each iteration, a new triangle is added on each side of the previous iteration, so the number of new triangles added in iteration n is:

The area of each new triangle added in an iteration is 1/9th of the area of each triangle added in the previous iteration, so the area of each triangle added in iteration n is:

where a_0 is the area of the original triangle. The total new area added in iteration n is therefore:

The total area of the snowflake after n iterations is:

Moreover, the limit of a Koch snowflake’s area is found to be:

Thus, the area of the Koch snowflake is 8/5 of the area of the original triangle. The above is just one example of how a fractal could be developed. Do not let the simplicity of the instructions or the familiarity of the shape deceive you – the mathematical complexity could span from the more straightforward Koch curves to the famously complicated Mandelbrot set (constructed by finding out for which c values that an initial complex input z_0 remains bounded after infinite iterations of the following quadratic function)

a fractal which, for four decades, mathematicians have struggled to map and understand.

The Mandelbrot Set.
Why do living beings develop fractals? The key is simplicity.
The beauty of fractals lies in how they are created by iterating only one or several operations. This means that only a small amount of genetic information is needed to encode and instruct the extensive continuous development of a structure.
Here is a general example. Imagine that we have a square block of cells, and we need a system which transports fluids such as oxygenated blood to every cell. While we could use a system such as that depicted by the left image, this system takes up too much of the block’s volume, which may, in a system such as the lungs, reduce the efficiency as the space in total is limited. However, a system such as that in the right image could reach out to most cells while taking the minimal volume of the block itself.
And here is a more specific example: the trachea of a fly (which, unlike humans, doesn't have lungs) that transfers oxygen to the entire body of the insect. In the fly’s embryo stage, the trachea is developed from a straight pipe throughout the body, from which surface a few lumps grow that would grow into branches.

A fly’s trachea.
The operation which governs where and how this branching process occurs is as follows: If the body cells near the trachea release a certain molecule, direction sensors in the trachea cells would detect it, and the trachea cells would move towards the molecule’s source, creating a primary branch. When the branch grows close enough to the source, the signal will become very strong, which causes tip cells to form and inhibits the branch from continuing lengthening. This branch will then continue branching off by the same operation.
In short, we could observe that, just like how one set of instructions govern the creation of the Koch curve, one gene set of instructions for this operation can control the branching of the entire trachea. This clearly shows the advantage of the principle of a fractal – minimising the information while maximising the result of the development – and occasionally creating elegant pictures such as that of the ice crystal or the Romanesco Broccoli.


It is worthy noting that although the layer of complexity brought by the molecule-releasing source cells (in the case of the fly’s trachea development) makes it seem that the process is not governed by a simple fractal equation, these sources have the role of counteracting the branching process according to how the external conditions trigger the molecule release. This means the process can automatically adapt to the environment without new genetic information – which is crucial for the survival of all living beings.
We could imagine that the human body would develop the bronchi and a tree would grow its branches using similar principles of the development of a fly’s trachea. In fact, the insights about pattern and structure that fractals have given us concern not only mathematics or biology, but also music and art. And that we are gifted with such a tool, which encapsulates both beauty and ingenious uses, is truly one of the best marvels of nature we could find.
Works Cited
Gunther, S. (2020). 14 Amazing Fractals Found in Nature. [online] Treehugger. Available at: https://www.treehugger.com/amazing-fractals-found-in-nature-4868776.
Larry Riddle, Agnes Scott College (2023). Koch Curve. [online] Larry Riddle, Agnes Scott College. Available at: https://larryriddle.agnesscott.org/ifs/kcurve/kcurve.htm.
NanoRooms (2023). The recursion formula behind life itself? [online] www.youtube.com. Available at: https://youtu.be/OMB1JLIdwNA?si=RKhTzp-zIbLdON3U [Accessed 28 Feb. 2024].
Wikipedia (2020). Koch snowflake. [online] Wikipedia. Available at: https://en.wikipedia.org/wiki/Koch_snowflake.
Xu, N., Pirraglia, C., Patel, U. and Monn Monn Myat (2012). Mechanisms of Lumen Development in Drosophila Tubular Organs. InTech eBooks. doi:https://doi.org/10.5772/36730.
.png)

Comments